 I am a regular reader of Ash Jogalekar’s blog Curious Wavefunction, but I found my way to his latest via the eclectic website 3 Quarks Daily, also highly recommended. I could not resist the title, “Mathematics, And The Excellence Of The Life It Brings”. The entirety of the post was about the mathematician Shing-Tung Yau’s recent memoir, The Shape of a Life, but Jogalekar’s introductory remarks about his personal involvement with mathematics stirred so many personal recollections of my own, that I thought I would provide an excerpt, followed by my own comments. Furthermore, he also addresses in passing the perennial question of whether math is invented or discovered.
I am a regular reader of Ash Jogalekar’s blog Curious Wavefunction, but I found my way to his latest via the eclectic website 3 Quarks Daily, also highly recommended. I could not resist the title, “Mathematics, And The Excellence Of The Life It Brings”. The entirety of the post was about the mathematician Shing-Tung Yau’s recent memoir, The Shape of a Life, but Jogalekar’s introductory remarks about his personal involvement with mathematics stirred so many personal recollections of my own, that I thought I would provide an excerpt, followed by my own comments. Furthermore, he also addresses in passing the perennial question of whether math is invented or discovered.
See Math and the Excellence of Life
(Update 8/9/2021) Jogalekar’s story about his embracing math and the effect Simmon’s topology book had on him is even more amazing than I thought. Throughout his younger years he had always been labeled “bad at math” and did poorly in school. But a teacher and Simmon’s book changed all that. He explains in a recent article in 3QuarksDaily, which I also provide here.
I can’t help singling out a section where he, too, extols the significance and importance of high school geometry (see my post “Down with Geometry”):
“… Purely through accident at this time, I had gotten my hands on a book on topology, a subject that I had become mildly interested in because of its deep connections to geometry; interestingly, while I was rather abysmal at algebra in school, I always did well with geometry because I was good at visualization. …
The topology book and the professor completely changed my outlook and saved me. I started doing well and tackling advanced topics and started to love math. I also got interested in physics and did well. Most importantly, I started appreciating the beauty of math. Over time I found that people interested in mathematics are generally of two kinds, although there’s some overlap: there are those who really enjoy mathematical puzzles and puzzle-like problems, relishing the raw process of problem-solving. Then there are others who simply enjoy the abstract nature of proofs and the connections between different topics: I am definitely part of this second group. In fact, another revelation I had was that most of the high school curriculum needed the students to be good at the former skill and had no appreciation of the latter, thus simply weeding out students like myself who wanted to understand the big picture and see the connections rather than just become adept at problem-solving.”
I confess I share this view and find it somewhat ironic that my website has devolved into a problem-solving source. I have tried to show the wider picture of fascinating connections, but that often takes more skill and time than I currently possess.
Post Views: 2,457
 The September 2019 Special Issue of Scientific American is a must read. Unfortunately it is behind a paywall, so you should purchase a copy at a store or digitally online. All the articles are fascinating and relevant, and address basic questions of epistemology—how do we know what we know? The first section, “Truth”, is the most pertinent to my thinking, as it covers three subjects I have been pondering for years.
The September 2019 Special Issue of Scientific American is a must read. Unfortunately it is behind a paywall, so you should purchase a copy at a store or digitally online. All the articles are fascinating and relevant, and address basic questions of epistemology—how do we know what we know? The first section, “Truth”, is the most pertinent to my thinking, as it covers three subjects I have been pondering for years.
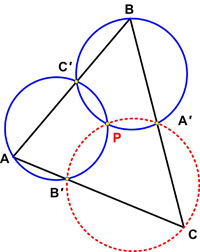 Here is yet another surprising result from Colin Hughes at Maths Challenge.
Here is yet another surprising result from Colin Hughes at Maths Challenge. This is a stimulating problem from the UKMT Senior Math Challenge for 2017. The additional problem “for investigation” is particularly challenging. (I have edited the problem slightly for clarity.)
This is a stimulating problem from the UKMT Senior Math Challenge for 2017. The additional problem “for investigation” is particularly challenging. (I have edited the problem slightly for clarity.) There is the famous chicken and the egg problem: If a chicken and a half can lay an egg and a half in a day and a half, how many eggs can three chickens lay in three days? Fibonacci 800 years ago in his book Liber Abaci (1202 AD) did not have exactly this problem (as far as I could find), but he posed its equivalent. And most likely the problem came even earlier from the Arabs. So we can essentially claim Fibonacci (or the Arabs) as the father of the chicken and egg problem. Here are three of Fibonacci’s actual problems:
There is the famous chicken and the egg problem: If a chicken and a half can lay an egg and a half in a day and a half, how many eggs can three chickens lay in three days? Fibonacci 800 years ago in his book Liber Abaci (1202 AD) did not have exactly this problem (as far as I could find), but he posed its equivalent. And most likely the problem came even earlier from the Arabs. So we can essentially claim Fibonacci (or the Arabs) as the father of the chicken and egg problem. Here are three of Fibonacci’s actual problems: This is another train puzzle by H. E. Dudeney. This one has some hairy arithmetic.
This is another train puzzle by H. E. Dudeney. This one has some hairy arithmetic. It is always fascinating to look at problems from the past. This one, given by Thomas Whiting himself, is over 200 years old from Whiting’s 1798 Mathematical, Geometrical, and Philosophical Delights:
It is always fascinating to look at problems from the past. This one, given by Thomas Whiting himself, is over 200 years old from Whiting’s 1798 Mathematical, Geometrical, and Philosophical Delights: I am a regular reader of Ash Jogalekar’s blog
I am a regular reader of Ash Jogalekar’s blog  This 2007 four-star problem from Colin Hughes at Maths Challenge is definitely a bit challenging.
This 2007 four-star problem from Colin Hughes at Maths Challenge is definitely a bit challenging. This is another intriguing problem from Presh Talwalkar.
This is another intriguing problem from Presh Talwalkar.