 This is a problem from the 629 AD work of Bhaskara I, a contemporary of Brahmagupta.
This is a problem from the 629 AD work of Bhaskara I, a contemporary of Brahmagupta.
“A fish is resting at the northeast corner of a rectangular pool. A heron standing at the northwest corner spies the fish. When the fish sees the heron looking at him he quickly swims towards the south (in a southwesterly direction rather than due south). When he reaches the south side of the pool, he has the unwelcome surprise of meeting the heron who has calmly walked due south along the side and turned at the southwest corner of the pool and proceeded due east, to arrive simultaneously with the fish on the south side. Given that the pool measures 12 units by 6 units, and that the heron walks as quickly as the fish swims, find the distance the fish swam.”
See Lunchtime at the Fish Pond for a solution.

 This is a problem from the 2000 Olymon (the Mathematics Olympiads Correspondence Program) for secondary students sponsored jointly by the Canadian Mathematical Society and the Mathematics Department of the University of Toronto.
This is a problem from the 2000 Olymon (the Mathematics Olympiads Correspondence Program) for secondary students sponsored jointly by the Canadian Mathematical Society and the Mathematics Department of the University of Toronto.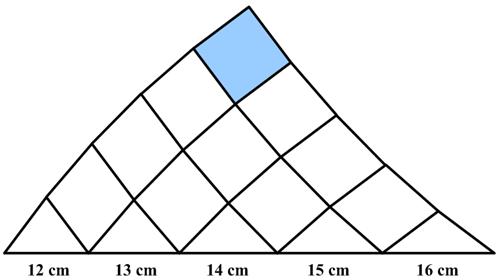 This is a
This is a 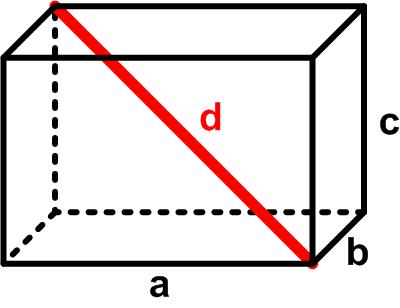 This is a slightly stimulating thought puzzle from Futility Closet.
This is a slightly stimulating thought puzzle from Futility Closet. This is a fairly straight-forward
This is a fairly straight-forward  This is a curious relation from the 2024 Math Calendar.
This is a curious relation from the 2024 Math Calendar.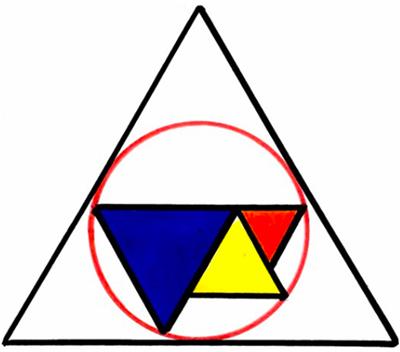 This is an old puzzle from Catriona Agg that I found on BL’s Math Games
This is an old puzzle from Catriona Agg that I found on BL’s Math Games 
 This is another problem from the c.100AD Chinese mathematical work, Jiǔ zhāng suàn shù (The Nine Chapters on the Mathematical Art) found at the MAA Convergence website Convergence.
This is another problem from the c.100AD Chinese mathematical work, Jiǔ zhāng suàn shù (The Nine Chapters on the Mathematical Art) found at the MAA Convergence website Convergence. This is an interesting problem from the 1966 Eureka magazine.
This is an interesting problem from the 1966 Eureka magazine.