 This is a fairly straight-forward logic puzzle from Alex Bellos’s Monday Puzzle in The Guardian.
This is a fairly straight-forward logic puzzle from Alex Bellos’s Monday Puzzle in The Guardian.
“When it comes to the world of mathematical puzzles, Hungary is a superpower. Not just because of the Rubik’s cube, the iconic toy invented by Ernő Rubik in 1974, but also because of its long history of maths outreach.
In 1894, Hungary staged the world’s first maths competition for teenagers, four decades before one was held anywhere else. 1894 also saw the launch of KöMaL, a Hungarian maths journal for secondary school pupils full of problems and tips on how to solve them. Both the competition and the journal have been running continuously since then, with only brief hiatuses during the two world wars.
This emphasis on developing young talent means that Hungarians are always coming up with puzzles designed to stimulate a love of mathematics. (It also explains why Hungary arguably produces, per capita, more top mathematicians than any other country.)
I asked Béla Bajnok, a Hungarian who is now director of American Mathematics Competitions, a series of competitions involving 300,000 students in the US, whether he knew of any puzzles that originated in Hungary. The first thing he said that came to mind was the ‘3-D logic puzzle’, a type of logic puzzle in which you work out the solution in a three dimensional box, rather than (as is the case with the standard version) in a two-dimensional grid. He said he had never seen this type of puzzle outside Hungary.
Below are two examples he created. You could solve these using an extended two dimensional grid. It’s more in the spirit of the question, however, to draw a three-dimensional one, like you are looking at three sides of a Rubik’s Cube.
Date night
Andy, Bill, Chris, and Daniel are out tonight with their dates, Emily, Fran, Gina, and Huong. We have the following information.
- Andy will go to the opera
- Bill will spend the evening with Emily,
- Chris would not want to go out with Gina,
- Fran will see a movie
- Gina will attend a workshop.
We also know that one couple will see an art exhibit. Who will go out with whom, and what will they do?”
See Date Night
Post Views: 707
 This is a slightly challenging problem from the 1993 American Invitational Mathematics Exam (AIME).
This is a slightly challenging problem from the 1993 American Invitational Mathematics Exam (AIME).
 This is a fairly straight-forward logic
This is a fairly straight-forward logic  Here is another Brainteaser from the Quantum math magazine.
Here is another Brainteaser from the Quantum math magazine. Here is a tricky little logarithm problem from the 2021 Math Calendar.
Here is a tricky little logarithm problem from the 2021 Math Calendar.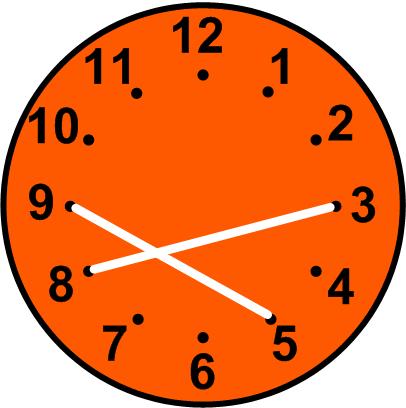 This is an imaginative puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings for 2012.
This is an imaginative puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings for 2012.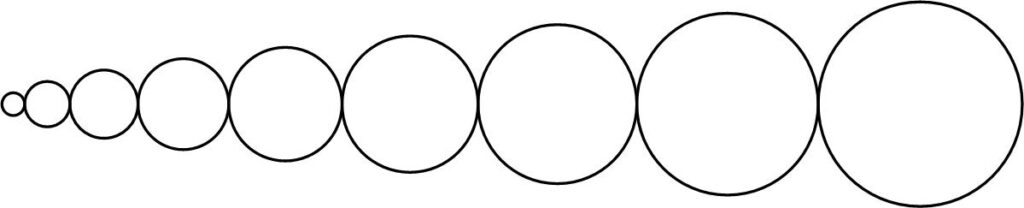
 I came across an interesting problem in the MathsJam Shout for February 2022.
I came across an interesting problem in the MathsJam Shout for February 2022.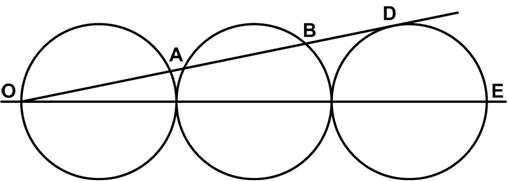 This is a straight-forward problem by Geoffrey Mott-Smith from 1954.
This is a straight-forward problem by Geoffrey Mott-Smith from 1954. This is another candle burning problem, presented by
This is another candle burning problem, presented by 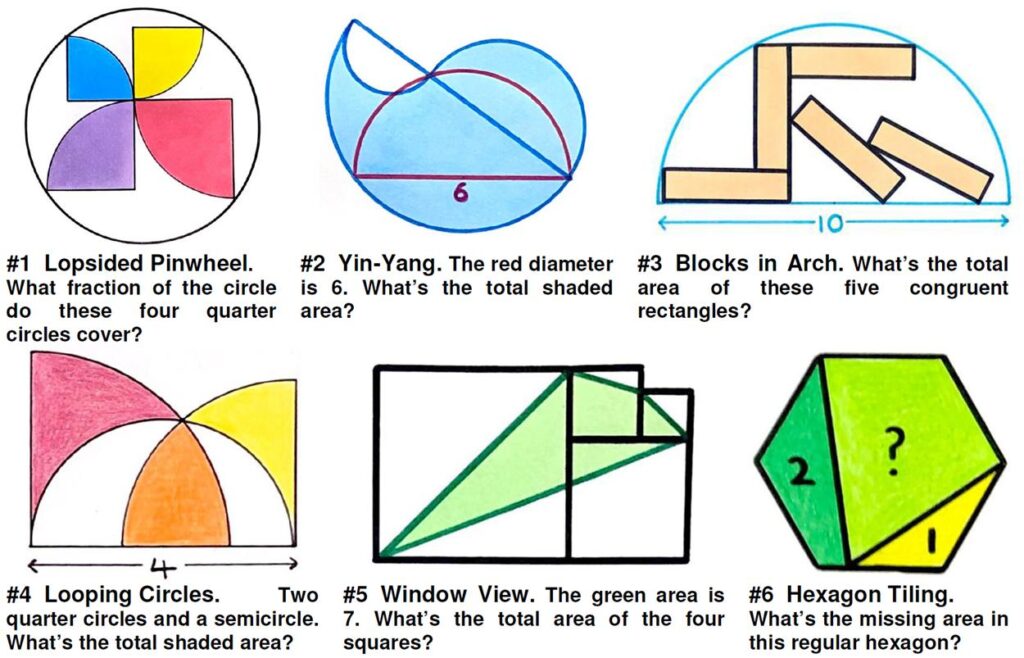 Here is yet another (belated) collection of beautiful geometric problems from Catriona Agg (née Shearer).
Here is yet another (belated) collection of beautiful geometric problems from Catriona Agg (née Shearer).