This is problem #25 from the UKMT 2014 Senior Challenge.
“Figure 1 shows a tile in the form of a trapezium [trapezoid], where a = 83⅓°. Several copies of the tile placed together form a symmetrical pattern, part of which is shown in Figure 2. The outer border of the complete pattern is a regular ‘star polygon’. Figure 3 shows an example of a regular ‘star polygon’.
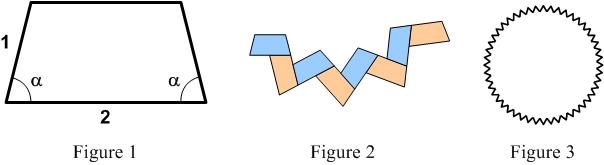 How many tiles are there in the complete pattern?
How many tiles are there in the complete pattern?
_____A_48_____B_54_____C_60_____D_66_____E_72”
See the Star Polygon Problem for solutions.

 Here is a problem from the UKMT Senior (17-18 year-old) Mathematics Challenge for 2012:
Here is a problem from the UKMT Senior (17-18 year-old) Mathematics Challenge for 2012: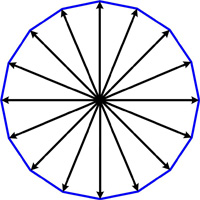 This is a fun problem from Mathematical Quickies (1967).
This is a fun problem from Mathematical Quickies (1967).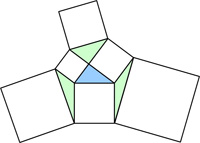 This is a problem from the UKMT Senior Challenge for 2001. (It has been slightly edited to reflect the colors I added to the diagram.)
This is a problem from the UKMT Senior Challenge for 2001. (It has been slightly edited to reflect the colors I added to the diagram.)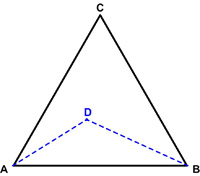 Catriona Shearer retweeted the following problem from Antonio Rinaldi
Catriona Shearer retweeted the following problem from Antonio Rinaldi  Another challenging problem from
Another challenging problem from 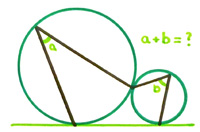 I really was trying to stop including
I really was trying to stop including  Since everyone by now who has any interest has gone directly to Catriona Shearer’s Twitter account for geometric puzzles, I was not going to include any more. But this one with its one-step solution is too fine to ignore and belongs with the “5 Problem” as one of the most elegant.
Since everyone by now who has any interest has gone directly to Catriona Shearer’s Twitter account for geometric puzzles, I was not going to include any more. But this one with its one-step solution is too fine to ignore and belongs with the “5 Problem” as one of the most elegant.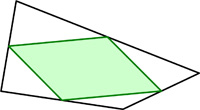 I came across this problem in Alfred Posamentier’s book, but I remember I had seen it a couple of places before and had never thought to solve it. At first, it seems like magic.
I came across this problem in Alfred Posamentier’s book, but I remember I had seen it a couple of places before and had never thought to solve it. At first, it seems like magic.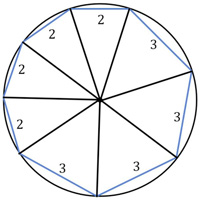 Here is a problem from the famous (infamous?) Putnam exam, presented by Presh Talwalkar. Needless to say, I did not solve it in 30 minutes—but at least I solved it (after making a blizzard of arithmetic and trigonometric errors).
Here is a problem from the famous (infamous?) Putnam exam, presented by Presh Talwalkar. Needless to say, I did not solve it in 30 minutes—but at least I solved it (after making a blizzard of arithmetic and trigonometric errors).