 I have been subverted again by a recent post by Ben Orlin, “Geometry Puzzles for a Winter’s Day,” which is another collection of Catriona Shearer’s geometric puzzles, this time her favorites for the month of November 2019 (which Orlin seems to have named himself). I often visit Orlin’s blog, “Math with Bad Drawings”, so it is hard to kick my addiction to Shearer’s puzzles if he keeps presenting collections. Her production volume is amazing, especially as she is able to maintain the quality that makes her problems so special.
I have been subverted again by a recent post by Ben Orlin, “Geometry Puzzles for a Winter’s Day,” which is another collection of Catriona Shearer’s geometric puzzles, this time her favorites for the month of November 2019 (which Orlin seems to have named himself). I often visit Orlin’s blog, “Math with Bad Drawings”, so it is hard to kick my addiction to Shearer’s puzzles if he keeps presenting collections. Her production volume is amazing, especially as she is able to maintain the quality that makes her problems so special.
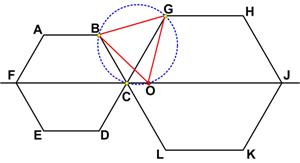
The Stained Glass puzzle generated some discussion about needed constraints to ensure a solution. Essentially, it was agreed to make explicit that the drawing had vertical and horizontal symmetry in the shapes, that is, flipping it horizontally or vertically kept the same shapes, though some of the colors might be swapped.

 This is truly an amazing result from Five Hundred Mathematical Challenges.
This is truly an amazing result from Five Hundred Mathematical Challenges.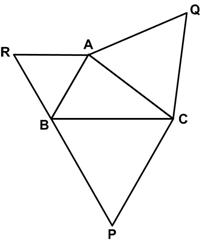 Here is another simple problem from Futility Closet.
Here is another simple problem from Futility Closet.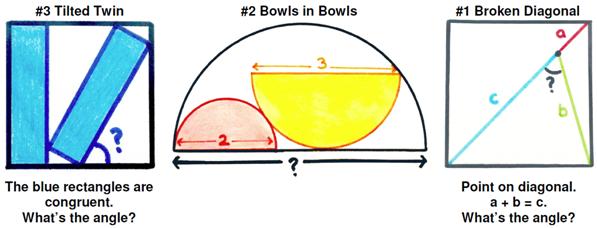
 This is another problem from the Math Challenges section of the 2000 Pi in the Sky Canadian math magazine for high school students.
This is another problem from the Math Challenges section of the 2000 Pi in the Sky Canadian math magazine for high school students.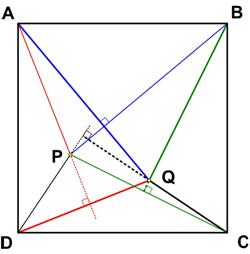 I found this problem from the Math Challenges section of the 2002 Pi in the Sky Canadian math magazine for high school students to be truly astonishing.
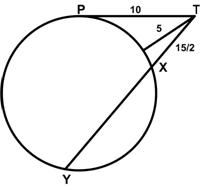
I found this problem from the Math Challenges section of the 2002 Pi in the Sky Canadian math magazine for high school students to be truly astonishing.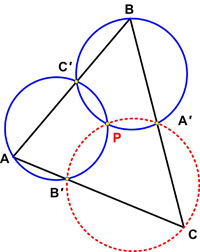 Here is yet another surprising result from Colin Hughes at Maths Challenge.
Here is yet another surprising result from Colin Hughes at Maths Challenge. This is from the UKMT Senior Challenge of 2004.
This is from the UKMT Senior Challenge of 2004.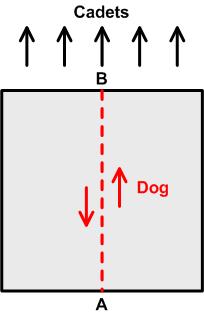 In my search for new problems I came across this one from Martin Gardner:
In my search for new problems I came across this one from Martin Gardner: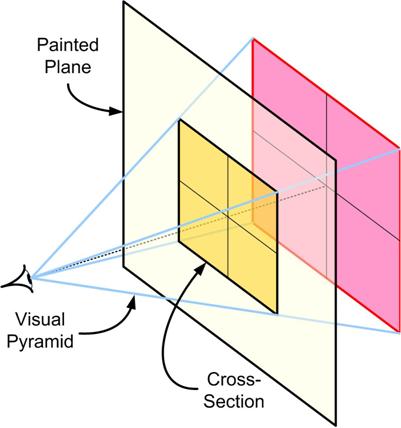 I was reading yet another book on the Scientific Revolution when I came across a discussion of the mathematical significance of the invention of perspective for painting in the 15th century Italian Renaissance. The main player in the saga was Leon Battista Alberti (1404 – 1472) and his tome De Pictura (On Painting) (1435-6), which contained the first mathematical presentation of perspective. Even though mathematics was advertised, it was not at the level of trigonometry I used in my post “
I was reading yet another book on the Scientific Revolution when I came across a discussion of the mathematical significance of the invention of perspective for painting in the 15th century Italian Renaissance. The main player in the saga was Leon Battista Alberti (1404 – 1472) and his tome De Pictura (On Painting) (1435-6), which contained the first mathematical presentation of perspective. Even though mathematics was advertised, it was not at the level of trigonometry I used in my post “