 This is a fairly extensive clock problem by Geoffrey Mott-Smith from 1954.
This is a fairly extensive clock problem by Geoffrey Mott-Smith from 1954.
“The clock shown in the illustration has just struck five. A number of things are going to happen in this next hour, and I am curious to know the exact times.
- At what time will the two hands coincide?
- At what time will the two hands first stand at right angles to each other?
- At one point the hands will stand at an angle of 30 degrees, the minute hand being before the hour hand. Then the former will pass the latter and presently make an angle of 60 degrees on the other side. How much time will elapse between these two events?”
See After Five O’Clock for solutions.

 This is a slightly challenging problem from Dan Griller.
This is a slightly challenging problem from Dan Griller.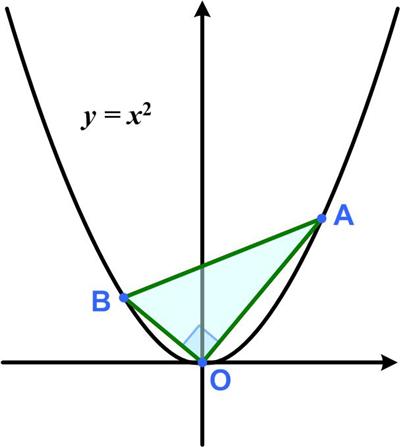 Since the changes in Twitter (now X), I have not been able to see the posts, not being a subscriber. But I noticed poking around that some twitter accounts were still viewable. However, like some demented aging octogenarian they had lost track of time, that is, instead of being sorted with the most recent post first, they showed a random scattering of posts from different times. So a current post could be right next to one several years ago. That is what I discovered with the now defunct MathsMonday site. I found a
Since the changes in Twitter (now X), I have not been able to see the posts, not being a subscriber. But I noticed poking around that some twitter accounts were still viewable. However, like some demented aging octogenarian they had lost track of time, that is, instead of being sorted with the most recent post first, they showed a random scattering of posts from different times. So a current post could be right next to one several years ago. That is what I discovered with the now defunct MathsMonday site. I found a  This essay is slightly tangential to my usual fare, but it is prompted by a most amazing video that convinced me that the impact of AI this time is not hype, but rather a real threat to our society. I found the
This essay is slightly tangential to my usual fare, but it is prompted by a most amazing video that convinced me that the impact of AI this time is not hype, but rather a real threat to our society. I found the 
 This is another puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings.
This is another puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings.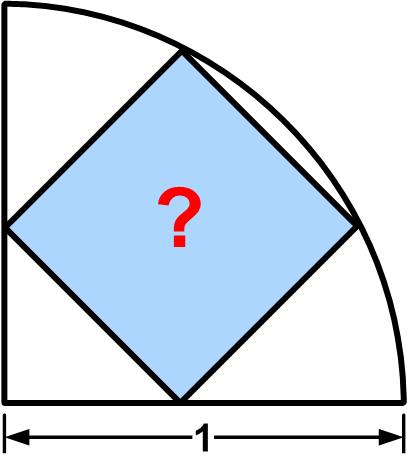 Another
Another  A prevalent theme of much of popular mathematical exposition and debates about mathematics education concerns how to interest a wider population in matters mathematical. For the most part I feel that essays that try to present the “beauty” of mathematics are doomed to failure, as are most discussions of esthetics. The underlying goal of such writing is a legitimate and laudable attempt to show the appeal of math. But I fear it succeeds only with those already converted. So is there another way?
A prevalent theme of much of popular mathematical exposition and debates about mathematics education concerns how to interest a wider population in matters mathematical. For the most part I feel that essays that try to present the “beauty” of mathematics are doomed to failure, as are most discussions of esthetics. The underlying goal of such writing is a legitimate and laudable attempt to show the appeal of math. But I fear it succeeds only with those already converted. So is there another way?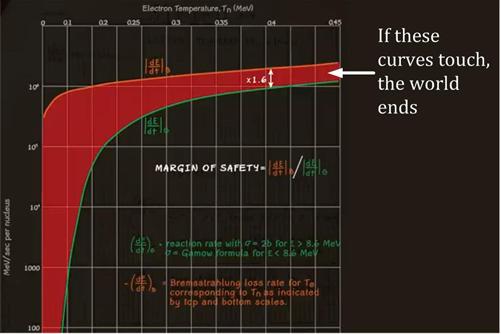 After a hiatus of four years, Stephen Welch is back with some timely videos at
After a hiatus of four years, Stephen Welch is back with some timely videos at  This is a problem from the 1987 American Invitational Mathematics Exam (AIME).
This is a problem from the 1987 American Invitational Mathematics Exam (AIME).