 This is another Brainteaser from the Quantum math magazine .
This is another Brainteaser from the Quantum math magazine .
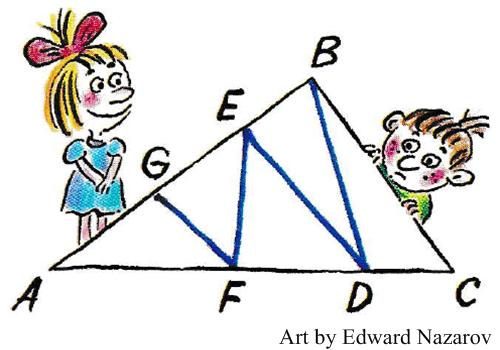
“How can a polygonal line BDEFG be drawn in a triangle ABC so that the five triangles obtained have the same area?”
I found this problem rather challenging, especially when I first tried to solve it analytically (using hyperbolas). Eventually I arrived at a procedure that would accomplish the result. (revised)
See the Equitable Slice Problem (revised)
(Update 9/22/2021) I goofed. I erroneously and foolishly thought Quantum had not solved the problem. Upon a closer reading I see what they were getting at and revised the posting.

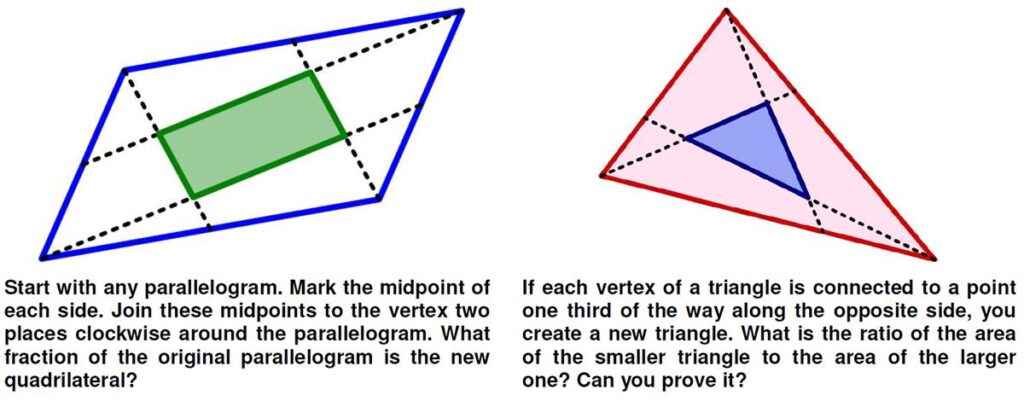 These two interesting problems were posed on MEI’s MathsMonday site on
These two interesting problems were posed on MEI’s MathsMonday site on  Here is another
Here is another  Here is another problem from the Sherlock Holmes puzzle book by Dr. Watson (aka Tim Dedopulos).
Here is another problem from the Sherlock Holmes puzzle book by Dr. Watson (aka Tim Dedopulos). This is a somewhat challenging math cryptogram in a slightly different guise from the Canadian Math Society’s magazine, Crux Mathematicorum.
This is a somewhat challenging math cryptogram in a slightly different guise from the Canadian Math Society’s magazine, Crux Mathematicorum.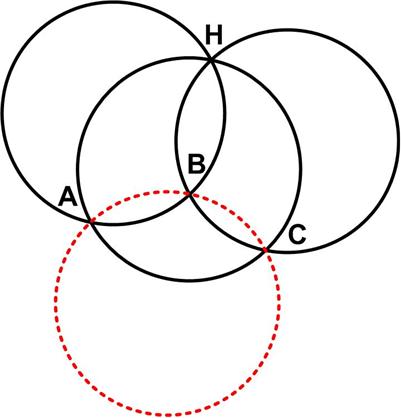 Here is a problem from the Quantum magazine, only this time from the “Challenges” section (these are expected to be a bit more difficult than the Brainteasers).
Here is a problem from the Quantum magazine, only this time from the “Challenges” section (these are expected to be a bit more difficult than the Brainteasers).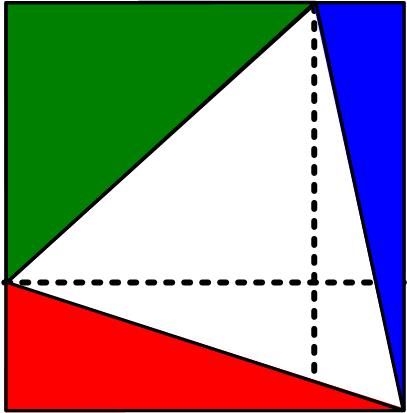 This simple-appearing problem is from the 17 August 2020 MathsMonday
This simple-appearing problem is from the 17 August 2020 MathsMonday 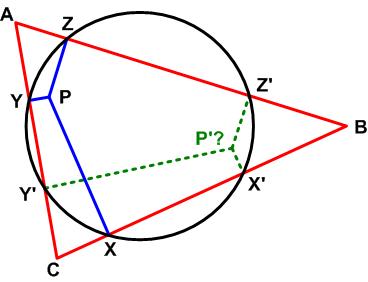 This is an interesting problem from the 1977 Canadian Math Society’s magazine, Crux Mathematicorum.
This is an interesting problem from the 1977 Canadian Math Society’s magazine, Crux Mathematicorum.