 The Futility Closet website had the following problem:
The Futility Closet website had the following problem:
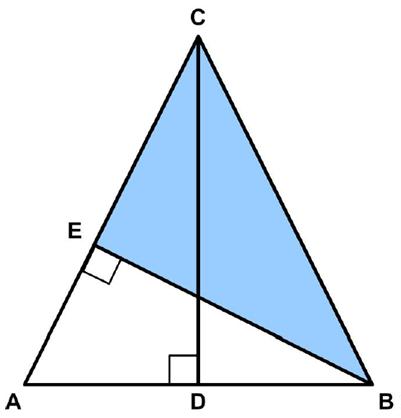
“In isosceles triangle ABC, CD = AB and BE is perpendicular to AC. Show that CEB is a 3-4-5 right triangle.”
See a Triangle Puzzle

 The Futility Closet website had the following problem:
The Futility Closet website had the following problem:
“In isosceles triangle ABC, CD = AB and BE is perpendicular to AC. Show that CEB is a 3-4-5 right triangle.”
See a Triangle Puzzle
 Here is another problem from the “Challenges” section of the Quantum magazine.
Here is another problem from the “Challenges” section of the Quantum magazine.
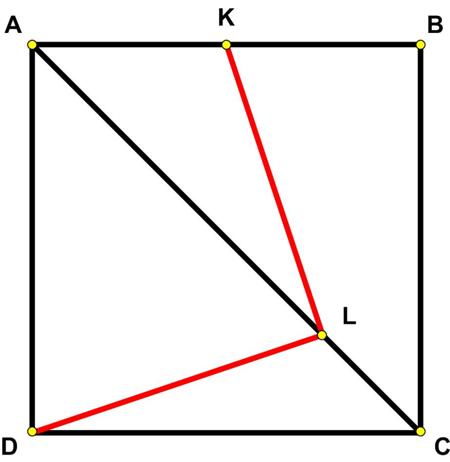
“Point L divides the diagonal AC of a square ABCD in the ratio 3:1, K is the midpoint of side AB. Prove that angle KLD is a right angle. (Y. Bogaturov)”
 This is a nice puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings.
This is a nice puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings.
“Two ants are on a cylindrical glass that is 5 centimetres in diameter. The ants are on opposite sides of the glass, 5 centimetres down from the glass’s rim. If both ants are on the outside of the glass, what is the shortest distance required for one ant to crawl to the other? What if one ant is on the outside of the glass and the other is on the inside?”
See the Ant Connection Problem for solution.
 This is a nifty problem from Presh Talwakar.
This is a nifty problem from Presh Talwakar.
“This is adapted from the 1994 Putnam, A2. Thanks to Nirman for the suggestion!
Let R be the region in the first quadrant bounded by the x-axis, the line y = x/2, and the ellipse x2/9 + y2 = 1. Let R‘ be the region in the first quadrant bounded by the y-axis, the line y = mx and the ellipse. Find the value of m such that R and R‘ have the same area.”
See the Putnam Ellipse Areas Problem for solution.
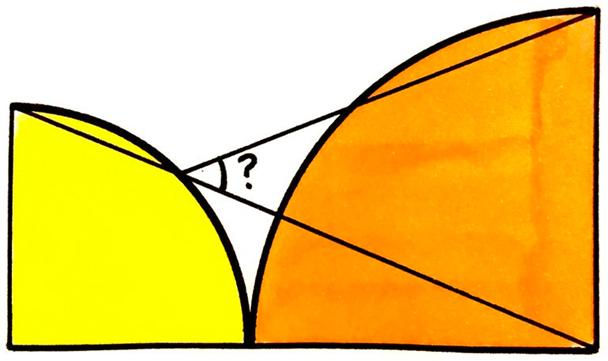 This is a Catriona Agg problem presented by itself, since it turned out to be the most challenging one I ever tried. Usually I can solve her problems in a few minutes or maybe hours, or sometimes days if they are especially challenging. But this problem has taken me weeks and I had to rely on a non-geometric argument. The problem is full of fascinating and unexpected relationships, but I couldn’t find a way to use them to prove the answer.
This is a Catriona Agg problem presented by itself, since it turned out to be the most challenging one I ever tried. Usually I can solve her problems in a few minutes or maybe hours, or sometimes days if they are especially challenging. But this problem has taken me weeks and I had to rely on a non-geometric argument. The problem is full of fascinating and unexpected relationships, but I couldn’t find a way to use them to prove the answer.
See the Curious Sunbeam Problem
(Update 5/5/2023, 7/22/2025) Alternative Solutions Continue reading
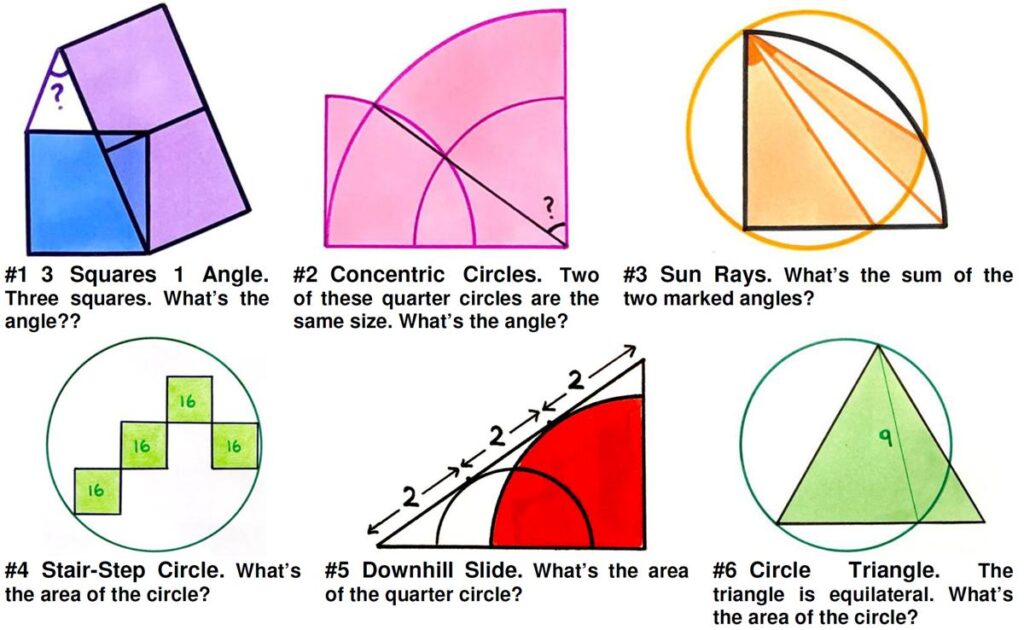 Here is yet another (belated) collection of beautiful geometric problems from Catriona Agg (née Shearer).
Here is yet another (belated) collection of beautiful geometric problems from Catriona Agg (née Shearer).
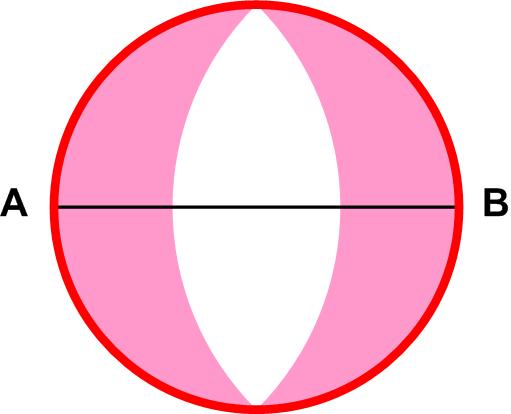 This is a straight-forward problem from the Scottish Mathematical Council (SMC) Senior Mathematics Challenge.
This is a straight-forward problem from the Scottish Mathematical Council (SMC) Senior Mathematics Challenge.
“A circle has radius 1 cm and AB is a diameter. Two circular arcs of equal radius are drawn with centres A and B. These arcs meet on the circle as shown. Calculate the shaded area.”
There are several possible approaches and the SMC offers two examples.
See the Moon Quarters Problem for solution.
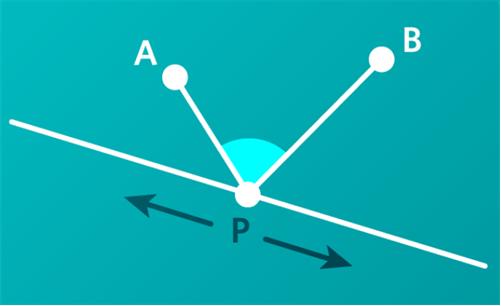 Here is a familiar puzzle from the Mathigon Puzzle Calendars for 2021.
Here is a familiar puzzle from the Mathigon Puzzle Calendars for 2021.
“Given a line and two points A and B, which point P on the line forms the largest angle APB?”
See the Max Angle Puzzle
 An excellent application of the solution to this puzzle can be found at Numberphile, where Ben Sparks explains an optimal rugby goal-kicking strategy.
An excellent application of the solution to this puzzle can be found at Numberphile, where Ben Sparks explains an optimal rugby goal-kicking strategy.
(Update 3/23/2023) Solution Construction
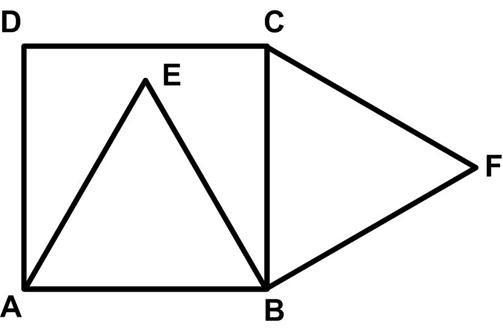 This is a fairly simple problem from Futility Closet, which is currently under a hiatus.
This is a fairly simple problem from Futility Closet, which is currently under a hiatus.
“Robert Bilinski proposed this problem in the April 2006 issue of Crux Mathematicorum. On square ABCD, two equilateral triangles are constructed, ABE internally and BCF externally, as shown. Prove that D, E, and F are collinear.”
See Line Work
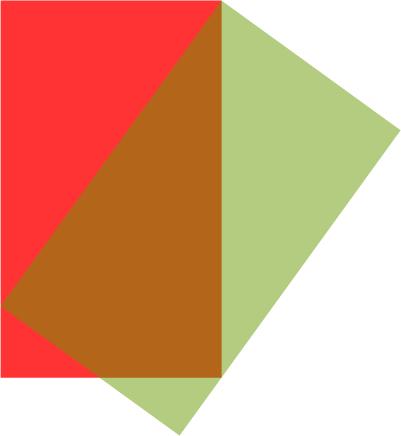 This is a nice puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings.
This is a nice puzzle from the Maths Masters team, Burkard Polster (aka Mathologer) and Marty Ross as part of their “Summer Quizzes” offerings.
“In the picture does the green rectangle cover more or less than half of the [congruent] red rectangle?”
It is evident from the problem solution that the two rectangles are the same, so I made it explicit.
See the Covering Rectangle Puzzle for solutions.