 In my search for new problems I came across this one from Martin Gardner:
In my search for new problems I came across this one from Martin Gardner:
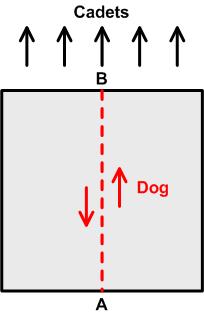
“A square formation of Army cadets, 50 feet on the side, is marching forward at a constant pace [see Figure]. The company mascot, a small terrier, starts at the center of the rear rank [position A in the illustration], trots forward in a straight line to the center of the front rank [position B], then trots back again in a straight line to the center of the rear. At the instant he returns to position A, the cadets have advanced exactly 50 feet. Assuming that the dog trots at a constant speed and loses no time in turning, how many feet does he travel?”
Gardner gives a follow-up problem that is virtually impossible:
“If you solve this problem, which calls for no more than a knowledge of elementary algebra, you may wish to tackle a much more difficult version proposed by the famous puzzlist Sam Loyd. Instead of moving forward and back through the marching cadets, the mascot trots with constant speed around the outside of the square, keeping as close as possible to the square at all times. (For the problem we assume that he trots along the perimeter of the square.) As before, the formation has marched 50 feet by the time the dog returns to point A. How long is the dog’s path?”
Answer.
See the Marching Cadets and Dog Problem for solutions.
![]() This problem from Colin Hughes at Maths Challenge is a most surprising result that takes a bit of tinkering to solve.
This problem from Colin Hughes at Maths Challenge is a most surprising result that takes a bit of tinkering to solve.
 This is another intriguing problem from Presh Talwalkar.
This is another intriguing problem from Presh Talwalkar. This interesting problem comes from Colin Hughes at the Maths Challenge website.
This interesting problem comes from Colin Hughes at the Maths Challenge website. A glutton for punishment I considered another Sam Loyd puzzle:
A glutton for punishment I considered another Sam Loyd puzzle: This is from the UKMT Senior Challenge of 2004.
This is from the UKMT Senior Challenge of 2004. In my search for new problems I came across this one from Martin Gardner:
In my search for new problems I came across this one from Martin Gardner: How many tiles are there in the complete pattern?
How many tiles are there in the complete pattern? This is another delightful H. E. Dudeney puzzle.
This is another delightful H. E. Dudeney puzzle. In a June Chalkdust
In a June Chalkdust  This is a tricky product problem from Alfred Posamentier which naturally has a slick solution—if you can think of it.
This is a tricky product problem from Alfred Posamentier which naturally has a slick solution—if you can think of it.