 Here is a challenging problem from the 1874 The Analyst.
Here is a challenging problem from the 1874 The Analyst.
“A cask containing a gallons of wine stands on another containing a gallons of water; they are connected by a pipe through which, when open, the wine can escape into the lower cask at the rate of c gallons per minute, and through a pipe in the lower cask the mixture can escape at the same rate; also, water can be let in through a pipe on the top of the upper cask at a like rate. If all the pipes be opened at the same instant, how much wine will be in the lower cask at the end of t minutes, supposing the fluids to mingle perfectly?
— Communicated by Artemas Martin, Mathematical Editor of Schoolday Magazine, Erie, Pennsylvania.”
I found the problem in Benjamin Wardhaugh’s book where he describes The Analyst:
“Beginning in 1874 and continuing as Annals of Mathematics from 1884 onward, The Analyst appeared monthly, published in Des Moines, Iowa, and was intended as “a suitable medium of communication between a large class of investigators and students in science, comprising the various grades from the students in our high schools and colleges to the college professor.” It carried a range of mathematical articles, both pure and applied, and a regular series of mathematical problems of varying difficulty: on the whole they seem harder than those in The Ladies’ Diary and possibly easier than the Mathematical Challenges in the extract after the next. Those given here appeared in the very first issue.”
I tailored my solution after the “Diluted Wine Puzzle”, though this problem was more complicated. Moreover, the final solution must pass from discreet steps to continuous ones.
There is a bonus problem in a later issue:
“19. Referring to Question 4, (No. 1): At what time will the lower cask contain the greatest quantity of wine?
—Communicated by Prof. Geo. R. Perkins.”
Answer.
See the Wine Into Water Problem for solutions.
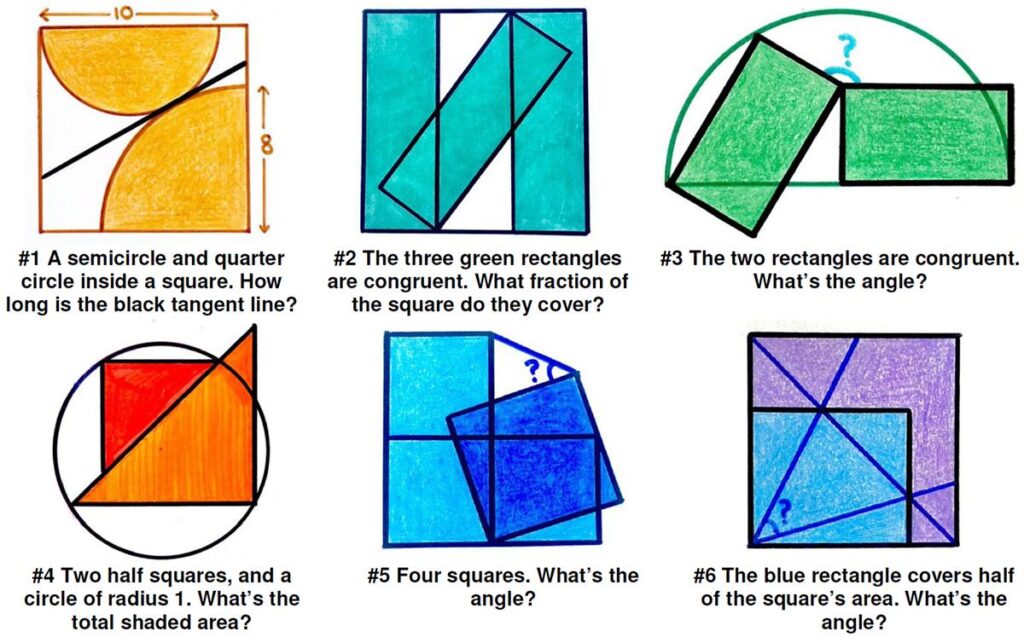 Here is yet another collection of beautiful geometric problems from Catriona Agg (née Shearer). For some reason I found these a bit more challenging than the previous ones. Some of them required more time to “see” the breakthrough.
Here is yet another collection of beautiful geometric problems from Catriona Agg (née Shearer). For some reason I found these a bit more challenging than the previous ones. Some of them required more time to “see” the breakthrough.
 Here is another challenging problem from the first issue of the 1874 The Analyst, which also appears in Benjamin Wardhaugh’s book.
Here is another challenging problem from the first issue of the 1874 The Analyst, which also appears in Benjamin Wardhaugh’s book. Here is a challenging problem from the 1874 The Analyst.
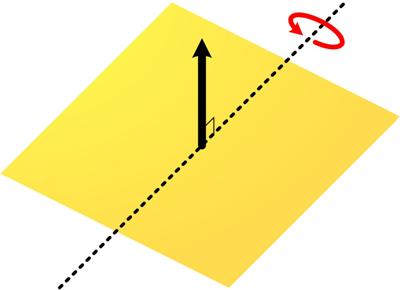
Here is a challenging problem from the 1874 The Analyst.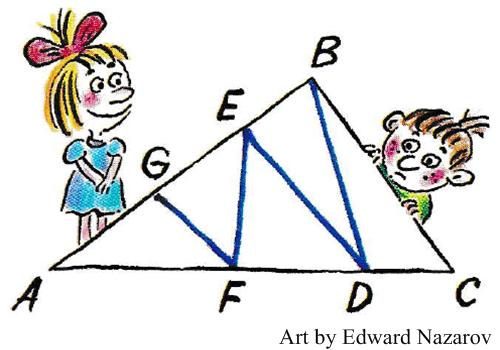 This is another Brainteaser from the Quantum math magazine .
This is another Brainteaser from the Quantum math magazine .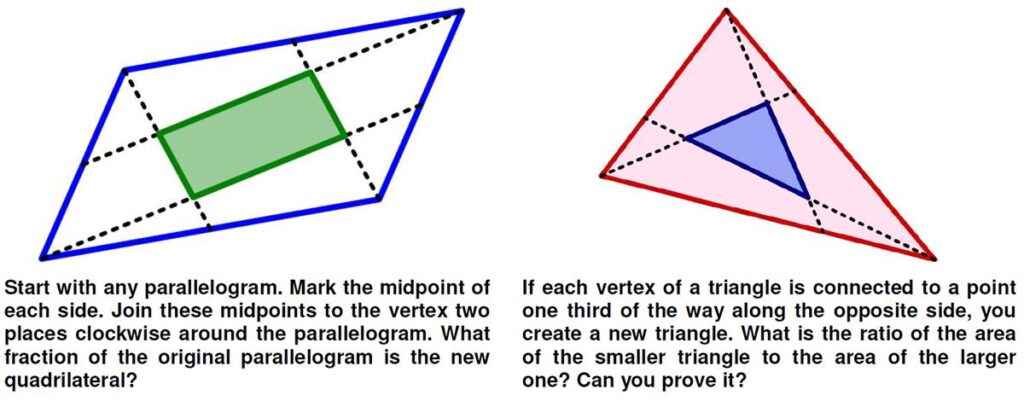 These two interesting problems were posed on MEI’s MathsMonday site on
These two interesting problems were posed on MEI’s MathsMonday site on  I thought it might be interesting to explore the mathematics of a common problem with a store-bought HO model train set that contains a collection of straight track segments and fixed-radius curved track segments that form a simple oval. Invariably an initial run of the train has it careening off the track when the train first meets the curved segment after running along the straight track segments.
I thought it might be interesting to explore the mathematics of a common problem with a store-bought HO model train set that contains a collection of straight track segments and fixed-radius curved track segments that form a simple oval. Invariably an initial run of the train has it careening off the track when the train first meets the curved segment after running along the straight track segments. Here is another
Here is another  Here is another problem from the Sherlock Holmes puzzle book by Dr. Watson (aka Tim Dedopulos).
Here is another problem from the Sherlock Holmes puzzle book by Dr. Watson (aka Tim Dedopulos). This is a somewhat challenging math cryptogram in a slightly different guise from the Canadian Math Society’s magazine, Crux Mathematicorum.
This is a somewhat challenging math cryptogram in a slightly different guise from the Canadian Math Society’s magazine, Crux Mathematicorum. Here is a problem from the Quantum magazine, only this time from the “Challenges” section (these are expected to be a bit more difficult than the Brainteasers).
Here is a problem from the Quantum magazine, only this time from the “Challenges” section (these are expected to be a bit more difficult than the Brainteasers).