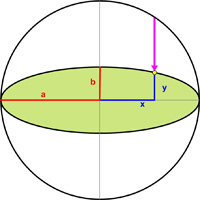
 I had been exploring how Kepler originally discovered his first two laws and became fascinated by what he did in his Astronomia Nova (1609), as presented by a number of researchers. Among the writers was A. E. L. Davis. She mentioned that the characterization of the ellipse that Kepler was using was the idea of a “compressed circle,” that is, a circle all of whose points were shrunk vertically by a constant amount towards a fixed diameter of the circle. I did not recall ever hearing this idea before and tried to track down its origin together with a proof — futilely, Davis’s references notwithstanding. I then tried to prove it myself. It was easy to do with analytic geometry. But in the spirit of the Kepler era (before the advent of Fermat’s and Descartes’s beginnings at fusing algebra and geometry) I tried to prove it solely within Euclid’s plane geometry. Some critical steps seemed to come from the great work of Apollonius of Perga (262-190 BC) on Conics. But for me a final elegant proof was not evident until 1822 when Dandelin employed his inscribed spheres. See Kepler’s Ellipse.
I had been exploring how Kepler originally discovered his first two laws and became fascinated by what he did in his Astronomia Nova (1609), as presented by a number of researchers. Among the writers was A. E. L. Davis. She mentioned that the characterization of the ellipse that Kepler was using was the idea of a “compressed circle,” that is, a circle all of whose points were shrunk vertically by a constant amount towards a fixed diameter of the circle. I did not recall ever hearing this idea before and tried to track down its origin together with a proof — futilely, Davis’s references notwithstanding. I then tried to prove it myself. It was easy to do with analytic geometry. But in the spirit of the Kepler era (before the advent of Fermat’s and Descartes’s beginnings at fusing algebra and geometry) I tried to prove it solely within Euclid’s plane geometry. Some critical steps seemed to come from the great work of Apollonius of Perga (262-190 BC) on Conics. But for me a final elegant proof was not evident until 1822 when Dandelin employed his inscribed spheres. See Kepler’s Ellipse.
In the process of exploring the compressed circle idea I acquired an immense appreciation and regard for Kepler and his perseverance in the face of the dominant paradigm of his era, namely, the 2000 year old idea that the celestial motions were all based on the most perfect motion of all, that of circles. The kinds of extremely laborious calculations he went through (just prior to the invention of logarithms by John Napier) were daunting, especially considering the trials he was undergoing in his personal life (trying to survive the religious destruction between Catholics and Protestants, along with defending his mother against charges of witchcraft).
 This is a stimulating problem from the UKMT Senior Math Challenge for 2017. The additional problem “for investigation” is particularly challenging. (I have edited the problem slightly for clarity.)
This is a stimulating problem from the UKMT Senior Math Challenge for 2017. The additional problem “for investigation” is particularly challenging. (I have edited the problem slightly for clarity.)
 When our daughter-in-law made wheat shocks as center-pieces for hers and our son’s fall-themed wedding reception, I naturally could not help pointing out the age-old observation that they represented a hyperboloid of one sheet. This was naturally greeted with the usual groans, but the thought stayed with me as I realized I had never proved this mathematically to myself. And so I did.
When our daughter-in-law made wheat shocks as center-pieces for hers and our son’s fall-themed wedding reception, I naturally could not help pointing out the age-old observation that they represented a hyperboloid of one sheet. This was naturally greeted with the usual groans, but the thought stayed with me as I realized I had never proved this mathematically to myself. And so I did.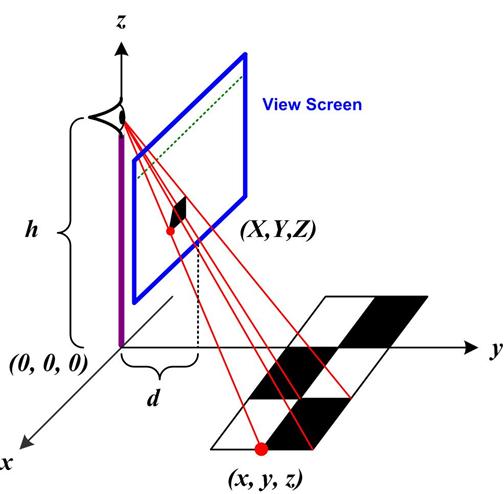 A number of recent puzzles have involved perspective views of objects. I had never really explored the idea of a perspective map in detail. So some of the properties associated with it always seemed a bit vague to me. I decided I would derive the mathematical equations for the perspective or projective map and see how its properties fell out from the equations. With this information in hand I then addressed some questions I had about the article “Dürer: Disguise, Distance, Disagreements, and Diagonals!” by Annalisa Crannell, Marc Frantz, and Fumiko Futamura concerning a controversy over Albrecht Dürer’s woodcut St. Jerome in His Study (1514). And finally, I read somewhere that a parabola under a perspective map becomes an ellipse, so I was able to show that as well. See the
A number of recent puzzles have involved perspective views of objects. I had never really explored the idea of a perspective map in detail. So some of the properties associated with it always seemed a bit vague to me. I decided I would derive the mathematical equations for the perspective or projective map and see how its properties fell out from the equations. With this information in hand I then addressed some questions I had about the article “Dürer: Disguise, Distance, Disagreements, and Diagonals!” by Annalisa Crannell, Marc Frantz, and Fumiko Futamura concerning a controversy over Albrecht Dürer’s woodcut St. Jerome in His Study (1514). And finally, I read somewhere that a parabola under a perspective map becomes an ellipse, so I was able to show that as well. See the  I had been exploring how Kepler originally discovered his first two laws and became fascinated by what he did in his Astronomia Nova (1609), as presented by a number of researchers. Among the writers was A. E. L. Davis. She mentioned that the characterization of the ellipse that Kepler was using was the idea of a “compressed circle,” that is, a circle all of whose points were shrunk vertically by a constant amount towards a fixed diameter of the circle. I did not recall ever hearing this idea before and tried to track down its origin together with a proof — futilely, Davis’s references notwithstanding. I then tried to prove it myself. It was easy to do with analytic geometry. But in the spirit of the Kepler era (before the advent of Fermat’s and Descartes’s beginnings at fusing algebra and geometry) I tried to prove it solely within Euclid’s plane geometry. Some critical steps seemed to come from the great work of Apollonius of Perga (262-190 BC) on Conics. But for me a final elegant proof was not evident until 1822 when Dandelin employed his inscribed spheres. See
I had been exploring how Kepler originally discovered his first two laws and became fascinated by what he did in his Astronomia Nova (1609), as presented by a number of researchers. Among the writers was A. E. L. Davis. She mentioned that the characterization of the ellipse that Kepler was using was the idea of a “compressed circle,” that is, a circle all of whose points were shrunk vertically by a constant amount towards a fixed diameter of the circle. I did not recall ever hearing this idea before and tried to track down its origin together with a proof — futilely, Davis’s references notwithstanding. I then tried to prove it myself. It was easy to do with analytic geometry. But in the spirit of the Kepler era (before the advent of Fermat’s and Descartes’s beginnings at fusing algebra and geometry) I tried to prove it solely within Euclid’s plane geometry. Some critical steps seemed to come from the great work of Apollonius of Perga (262-190 BC) on Conics. But for me a final elegant proof was not evident until 1822 when Dandelin employed his inscribed spheres. See 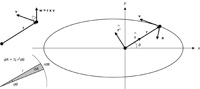 Years ago (1963) I got the paperback The Calculus:A Genetic Approach, by Otto Toeplitz, which presented the basic ideas of the differential and integral calculus from a historical point of view. One thing Toeplitz did at the end of his book that I had not seen in other texts was to show the equivalence of Kepler’s Laws and Newton’s Law of Gravity. (Since 1963 David Bressoud has developed this theme in his excellent 1991 text.) I thought I would try to emulate Toeplitz’s approach with more modern notation (vectors) and arguments in hopes of extracting the essential ideas from the clutter.
Years ago (1963) I got the paperback The Calculus:A Genetic Approach, by Otto Toeplitz, which presented the basic ideas of the differential and integral calculus from a historical point of view. One thing Toeplitz did at the end of his book that I had not seen in other texts was to show the equivalence of Kepler’s Laws and Newton’s Law of Gravity. (Since 1963 David Bressoud has developed this theme in his excellent 1991 text.) I thought I would try to emulate Toeplitz’s approach with more modern notation (vectors) and arguments in hopes of extracting the essential ideas from the clutter.