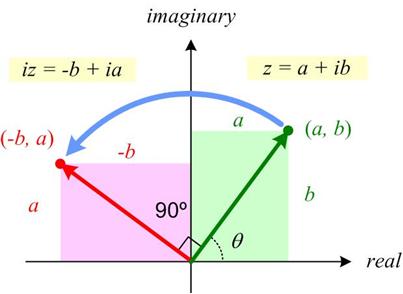
 This may be a futile attempt at an elementary introduction to complex variables by emphasizing their geometric properties. The elementary part is probably undermined by an initial discussion of field extensions and a necessary reference to trigonometry. Hopefully, the suppression of the explicit use of complex powers of Euler’s constant e until the very end will allow the geometric ideas to have center stage. A primary goal of the essay is to realize that complex polynomials involve sums of circles in the plane. The image of real polynomials as wavy curves in the plane is misleading for an understanding of complex behavior. See Complex Numbers – Geometric Viewpoint.
This may be a futile attempt at an elementary introduction to complex variables by emphasizing their geometric properties. The elementary part is probably undermined by an initial discussion of field extensions and a necessary reference to trigonometry. Hopefully, the suppression of the explicit use of complex powers of Euler’s constant e until the very end will allow the geometric ideas to have center stage. A primary goal of the essay is to realize that complex polynomials involve sums of circles in the plane. The image of real polynomials as wavy curves in the plane is misleading for an understanding of complex behavior. See Complex Numbers – Geometric Viewpoint.
Tag Archives: calculus
Polygon Altitude Problems I
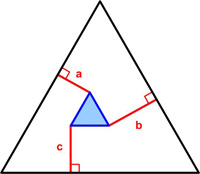 I found this collection of related problems by James Tanton on Twitter. Even though all these problems do not involve perpendiculars, they have a common solution approach – a sort of theme and variations idea. In a later tweet Tanton refers to a Viviani Theorem associated with these types of problems. I did not recall that theorem explicitly or by name. I also have not looked it up yet, in order to solve these problems on my own. I am guessing there is a more classical Euclidean geometry proof, but I like my vector approach for its clarity. I also throw in a bit a calculus at the end for fun. See Polygon Altitude Problems I
I found this collection of related problems by James Tanton on Twitter. Even though all these problems do not involve perpendiculars, they have a common solution approach – a sort of theme and variations idea. In a later tweet Tanton refers to a Viviani Theorem associated with these types of problems. I did not recall that theorem explicitly or by name. I also have not looked it up yet, in order to solve these problems on my own. I am guessing there is a more classical Euclidean geometry proof, but I like my vector approach for its clarity. I also throw in a bit a calculus at the end for fun. See Polygon Altitude Problems I
Perspective Map
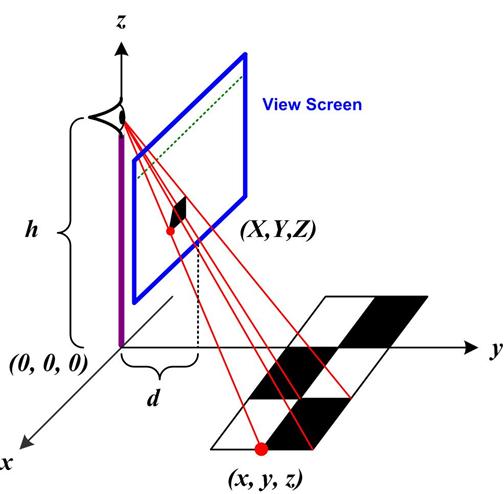 A number of recent puzzles have involved perspective views of objects. I had never really explored the idea of a perspective map in detail. So some of the properties associated with it always seemed a bit vague to me. I decided I would derive the mathematical equations for the perspective or projective map and see how its properties fell out from the equations. With this information in hand I then addressed some questions I had about the article “Dürer: Disguise, Distance, Disagreements, and Diagonals!” by Annalisa Crannell, Marc Frantz, and Fumiko Futamura concerning a controversy over Albrecht Dürer’s woodcut St. Jerome in His Study (1514). And finally, I read somewhere that a parabola under a perspective map becomes an ellipse, so I was able to show that as well. See the Perspective Map.
A number of recent puzzles have involved perspective views of objects. I had never really explored the idea of a perspective map in detail. So some of the properties associated with it always seemed a bit vague to me. I decided I would derive the mathematical equations for the perspective or projective map and see how its properties fell out from the equations. With this information in hand I then addressed some questions I had about the article “Dürer: Disguise, Distance, Disagreements, and Diagonals!” by Annalisa Crannell, Marc Frantz, and Fumiko Futamura concerning a controversy over Albrecht Dürer’s woodcut St. Jerome in His Study (1514). And finally, I read somewhere that a parabola under a perspective map becomes an ellipse, so I was able to show that as well. See the Perspective Map.
(Update 7/1/2019) Continue reading
Hossenfelder Stagnation Problem
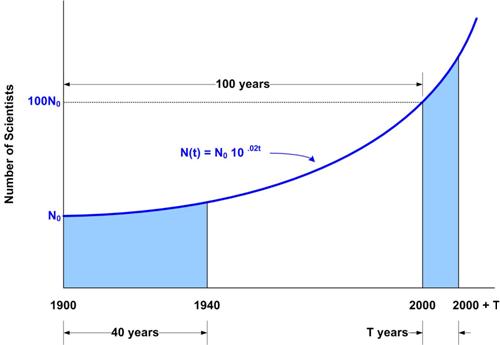 Sabine Hossenfelder wrote an excellent blog posting about the growing awareness that outstanding scientific problems are not getting solved at the same rate as in the past. Her whole article is worth a read, as are all her postings, but this latest contained a mathematical statement that warranted justification. For scientists “How much working time starting today corresponds to, say, 40 years working time starting 100 years ago. Have a guess! Answer: About 14 months.” See Hossenfelder Stagnation Problem.
Sabine Hossenfelder wrote an excellent blog posting about the growing awareness that outstanding scientific problems are not getting solved at the same rate as in the past. Her whole article is worth a read, as are all her postings, but this latest contained a mathematical statement that warranted justification. For scientists “How much working time starting today corresponds to, say, 40 years working time starting 100 years ago. Have a guess! Answer: About 14 months.” See Hossenfelder Stagnation Problem.
Triangular Boundary Problem
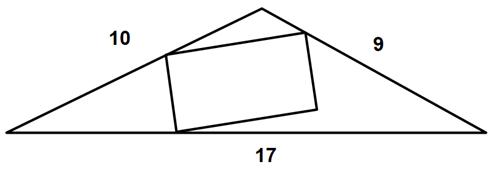 This problem comes from the defunct Wall Street Journal Varsity Math Week collection.
This problem comes from the defunct Wall Street Journal Varsity Math Week collection.
“The coach then shows the team the diagram to the left and asks: What is the maximum area of a rectangle contained entirely within a triangle with sides of 9, 10 and 17?”
I changed the numbers a bit to make my calculations easier, but left the problem otherwise unchanged. When I checked the Varsity Math Week solution, I saw they used a simplifying formula that I could not remember. I also believed their solution left out a justification for the maximal area. Besides an intuitive solution for this, I also included a calculus version.
See the Triangular Boundary Problem for solutions.
Kepler’s Laws and Newton’s Laws
 Years ago (1963) I got the paperback The Calculus:A Genetic Approach, by Otto Toeplitz, which presented the basic ideas of the differential and integral calculus from a historical point of view. One thing Toeplitz did at the end of his book that I had not seen in other texts was to show the equivalence of Kepler’s Laws and Newton’s Law of Gravity. (Since 1963 David Bressoud has developed this theme in his excellent 1991 text.) I thought I would try to emulate Toeplitz’s approach with more modern notation (vectors) and arguments in hopes of extracting the essential ideas from the clutter.
Years ago (1963) I got the paperback The Calculus:A Genetic Approach, by Otto Toeplitz, which presented the basic ideas of the differential and integral calculus from a historical point of view. One thing Toeplitz did at the end of his book that I had not seen in other texts was to show the equivalence of Kepler’s Laws and Newton’s Law of Gravity. (Since 1963 David Bressoud has developed this theme in his excellent 1991 text.) I thought I would try to emulate Toeplitz’s approach with more modern notation (vectors) and arguments in hopes of extracting the essential ideas from the clutter.
A by-product of this effort was to reveal strongly the different paths that physics and mathematics follow in understanding physical reality. The mystery is that the mathematics ends up describing the physics so well. I will return to this theme a number of times in other posts. See Kepler’s Laws and Newton’s Laws.
