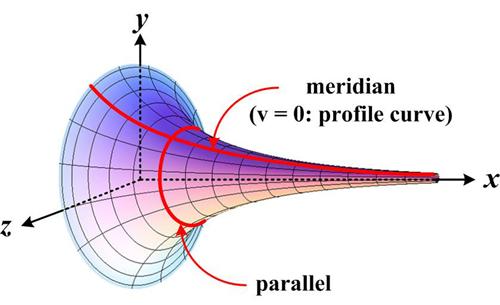
 This essay began as an effort to prove Tanya Khovanova’s statement in her article “The Annoyance of Hyperbolic Surfaces” that her crocheted hyperbolic surface had constant (negative) curvature. I discussed Khovanova’s article in my previous essay “Exponential Yarn”. What I thought would be a fairly straight-forward exercise turned into a more concerted effort as I concluded that her crocheted surface did not have constant curvature. However, I found additional references that supported her statement, so I was becoming quite confused. I looked at other, similar surfaces to try to understand the whole curvature situation. This involved a lot of tedious computations (with my usual plethora of mistakes) that proved most challenging. But then I realized where I had gone astray. To cover my ignorance I claimed my error stemmed from a subtle misunderstanding. Herewith is a presentation of what I found. See Bugles, Trumpets, and Beltrami.
This essay began as an effort to prove Tanya Khovanova’s statement in her article “The Annoyance of Hyperbolic Surfaces” that her crocheted hyperbolic surface had constant (negative) curvature. I discussed Khovanova’s article in my previous essay “Exponential Yarn”. What I thought would be a fairly straight-forward exercise turned into a more concerted effort as I concluded that her crocheted surface did not have constant curvature. However, I found additional references that supported her statement, so I was becoming quite confused. I looked at other, similar surfaces to try to understand the whole curvature situation. This involved a lot of tedious computations (with my usual plethora of mistakes) that proved most challenging. But then I realized where I had gone astray. To cover my ignorance I claimed my error stemmed from a subtle misunderstanding. Herewith is a presentation of what I found. See Bugles, Trumpets, and Beltrami.
(Update 4/6/2019) Continue reading

 Tanya Khovanova’s recent blog post “The Annoyance of Hyperbolic Surfaces” about crocheting a hyperbolic surface added to the numerous examples of such activity, usually from knitting. Somehow this post caught my attention, in particular about the exponential growth of each added row and the fact that the resulting “surface” had constant negative curvature. I explored the exponential growth in this article and saved the mathematical exploration of the constant negative curvature for a later essay. See
Tanya Khovanova’s recent blog post “The Annoyance of Hyperbolic Surfaces” about crocheting a hyperbolic surface added to the numerous examples of such activity, usually from knitting. Somehow this post caught my attention, in particular about the exponential growth of each added row and the fact that the resulting “surface” had constant negative curvature. I explored the exponential growth in this article and saved the mathematical exploration of the constant negative curvature for a later essay. See 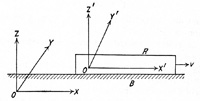 Over the years one of the subjects I return to periodically to study is Einstein’s Theory of Relativity, both the Special and General theories. Interest in the Special Theory focused on the derivation of the Lorentz transformations (or contractions). Why did objects appear with different lengths and clocks run at different speeds for observers moving relative to one another? Early on (late 60s) I came across a great explanation in the 1923 book by C. P. Steinmetz. He derived it from two general assumptions of special relativity: (1) that all motion is relative, the motion of the railway train relative to the track being the same as the motion of the track relative to the train, and (2) that the laws of nature, and thus the velocity of light, are the same everywhere. I did not follow his derivation completely, so I produced my own, which I will give here. See the
Over the years one of the subjects I return to periodically to study is Einstein’s Theory of Relativity, both the Special and General theories. Interest in the Special Theory focused on the derivation of the Lorentz transformations (or contractions). Why did objects appear with different lengths and clocks run at different speeds for observers moving relative to one another? Early on (late 60s) I came across a great explanation in the 1923 book by C. P. Steinmetz. He derived it from two general assumptions of special relativity: (1) that all motion is relative, the motion of the railway train relative to the track being the same as the motion of the track relative to the train, and (2) that the laws of nature, and thus the velocity of light, are the same everywhere. I did not follow his derivation completely, so I produced my own, which I will give here. See the 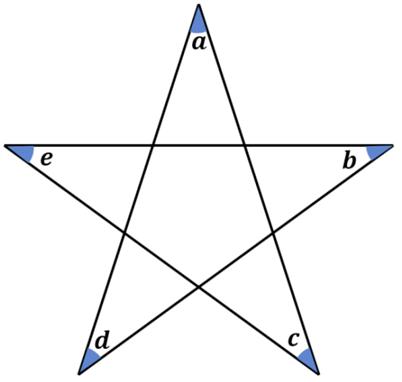 This problem posted by Presh Talwalkar offers a variety of solutions, but I didn’t quite see my favorite approach for such problems. So I thought I would add it to the mix.
This problem posted by Presh Talwalkar offers a variety of solutions, but I didn’t quite see my favorite approach for such problems. So I thought I would add it to the mix.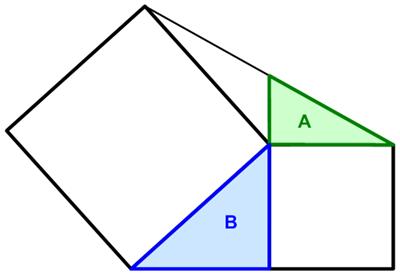 This was a nice geometric problem from Poo-Sung Park
This was a nice geometric problem from Poo-Sung Park  This is another UKMT Senior Challenge problem, this time from 2006.
This is another UKMT Senior Challenge problem, this time from 2006. I came across the following problem from an Italian high school exam on the British
I came across the following problem from an Italian high school exam on the British 
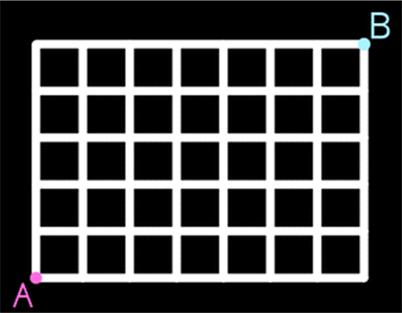 Normally I don’t care for combinatorial problems, but this problem from
Normally I don’t care for combinatorial problems, but this problem from  The following is a famous problem of Bachet as recounted by Heinrich Dörrie in his book 100 Great Problems of Elementary Mathematics:
The following is a famous problem of Bachet as recounted by Heinrich Dörrie in his book 100 Great Problems of Elementary Mathematics: This is a fun little problem from the United Kingdom Mathematics Trust (UKMT) Senior Math Challenge of 2008.
This is a fun little problem from the United Kingdom Mathematics Trust (UKMT) Senior Math Challenge of 2008.