 Here is another problem from the 2020 Math Calendar.
Here is another problem from the 2020 Math Calendar.
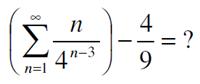
As a hint, recall that all the answers are integer days of the month. And the solution employs a technique familiar to these pages.
See Autumn Sum for a solution.

 Here is another problem from the 2020 Math Calendar.
Here is another problem from the 2020 Math Calendar.
As a hint, recall that all the answers are integer days of the month. And the solution employs a technique familiar to these pages.
See Autumn Sum for a solution.
 The following problem comes from a 1961 exam set collected by Ed Barbeau of the University of Toronto. The discontinued exams (by 2003) were for 5th year Ontario high school students seeking entrance and scholarships for the second year at a university.
The following problem comes from a 1961 exam set collected by Ed Barbeau of the University of Toronto. The discontinued exams (by 2003) were for 5th year Ontario high school students seeking entrance and scholarships for the second year at a university.
“If sn denotes the sum of the first n natural numbers, find the sum of the infinite series
![]() .”
.”
Unfortunately, the “Grade XIII” exam problem sets were not provided with answers, so I have no confirmation for my result. There may be a cunning way to manipulate the series to get a solution, but I could not see it off-hand. So I employed my tried and true power series approach to get my answer. It turned out to be power series manipulations on steroids, so there must be a simpler solution that does not use calculus. I assume the exams were timed exams, so I am not sure how a harried student could come up with a quick solution. I would appreciate any insights into this.
See Serious Series for a solution.
(Update 1/18/2021, 9/6/2024) Other Solutions
Continue reading
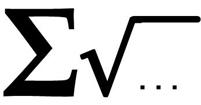 The craziness of manipulating radicals strikes again. This 2006 four-star problem from Colin Hughes at Maths Challenge is really astonishing, though it takes the right key to unlock it.
The craziness of manipulating radicals strikes again. This 2006 four-star problem from Colin Hughes at Maths Challenge is really astonishing, though it takes the right key to unlock it.
“Problem Consider the following sequence:
For which values of [positive integer] n is S(n) rational?”
See Amazing Radical Sum for a solution.
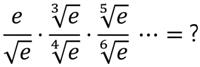 This is a delightful and surprising problem from Presh Talwalkar.
This is a delightful and surprising problem from Presh Talwalkar.
“This puzzle was created by a MindYourDecisions fan in India. What is the value of the infinite product? The numerators are the odd nth roots of [Euler’s constant] e and the denominators are even nth roots of e.”
See Euler Magic for a solution.
 If you will pardon the pun, this is a diabolical problem from the collection Five Hundred Mathematical Challenges.
If you will pardon the pun, this is a diabolical problem from the collection Five Hundred Mathematical Challenges.
“Problem 5. Calculate the sum![]()
__________
It has a non-calculus solution, but that involves a bunch of manipulations that were not that evident to me, or at least I doubt if I could have come up with them. I was able to reframe the problem using one of my favorite approaches, power series (or polynomials). The calculations are a bit hairy in any case, but I was impressed that my method worked at all.
See the Number of the Beast for solutions.
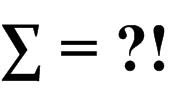 This is another stimulating math problem from Colin Hughes’s Maths Challenge website (mathschallenge.net).
This is another stimulating math problem from Colin Hughes’s Maths Challenge website (mathschallenge.net).
“Problem
Find the exact value of the following infinite series:
1/2! + 2/3! + 3/4! + 4/5! + …”
See the Unexpected Sum for solutions.

This is from the UKMT Senior Challenge of 1999.
What is the sum to infinity of the convergent series![]()
A_7/4_____B_2_____C_√5_____D_9/4_____E_7/3”
See Fibonacci Fandango for a solution.
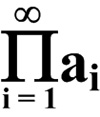 This is a challenging problem from Mathematical Quickies (1967).
This is a challenging problem from Mathematical Quickies (1967).
“Evaluate the infinite product:![]() ”
”
I came up with a motivated solution using some standard techniques from calculus. Mathematical Quickies had a solution that did not employ calculus, but one which I felt used unmotivated tricks.
See the Infinite Product Problem for solutions.
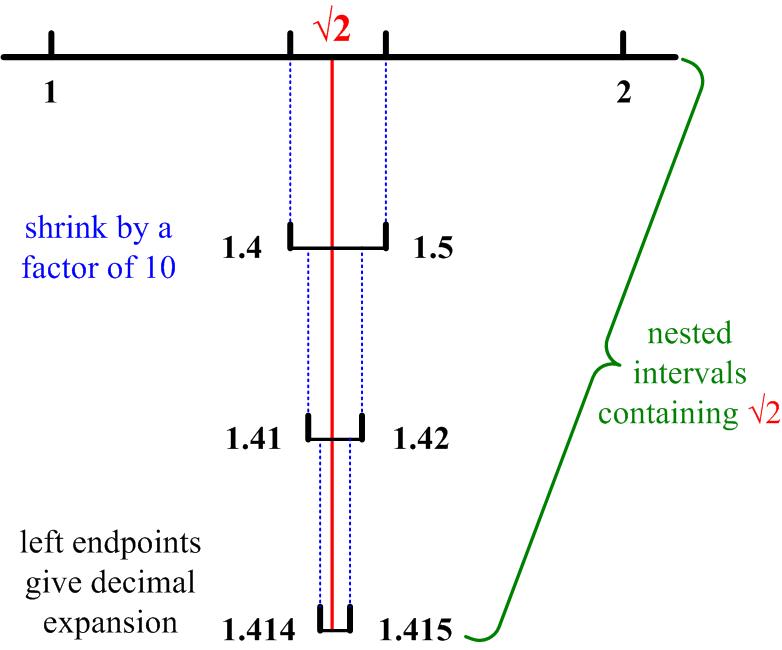 Probably the most satisfying article I have put together is a recent one on point set topology. An old friend of mine, who studied math and physics in college but ended up getting a doctorate in English, asked me, what was topology? Knowing that there were two main branches of topology (general or point set topology and algebraic topology), I chose to describe point set topology first, especially since it was what I was most familiar with and had worked with most in my graduate work.
Probably the most satisfying article I have put together is a recent one on point set topology. An old friend of mine, who studied math and physics in college but ended up getting a doctorate in English, asked me, what was topology? Knowing that there were two main branches of topology (general or point set topology and algebraic topology), I chose to describe point set topology first, especially since it was what I was most familiar with and had worked with most in my graduate work.
The essay turned out to have a surprising structure more like a musical theme and variations. The theme was the geometric series. I found it to be a wonderful medium to show the evolution of ideas (acting as variations) from the early Greeks (Zeno’s Paradoxes) through the development of calculus, decimal expansions of real numbers, to power series, metric spaces, and finally general topologies.
There was an additional benefit to this series of transformations of an initial idea: one of the major aspects of true mathematics became evident, namely, the extension of an idea into new territories that reveal unexpected connections to other forms of mathematics. Treating complicated functions as points in a topological space was a wonderful idea developed over the end of the 19th and beginning of the 20th centuries and became the basis of the field of functional analysis. See Point Set Topology (revised).
(Update 6/3/2021) Slightly revised version.
I happened to review this article and noticed I made a mistake in my integration example. I have no idea what I was thinking at the time, so I corrected it. As I reviewed the rest of the article, I noticed a bunch of “typos” that would make the text confusing, so I corrected those as well. And finally I rephrased wording in a couple of places to try to make things clearer.