Tag Archives: Dan Griller

Two Containers Mixing Puzzle

This is a slightly different type of a mixture problem from Dan Griller.

“Two containers A and B sit on a table, partially filled with water.  First, 40% of the water in A is poured into B, which completely fills it.  Then 75% of the water in B is poured into A, which completely fills it.  80% of the water in A is poured into B, which completely fills it.  Calculate the ratio of the capacity of container A to the capacity of container B, and the fraction of container A that was occupied by water at the start.”

Answer.

See the Two Containers Mixing Puzzle for solution.

Language Students Puzzle

This is a slightly challenging problem from Dan Griller.

“Every pupil at the Euler Academy studies French or Spanish.  At the start of the year, one third of the French students also studied Spanish, and 2 fifths of the Spanish students also studied French.  After one term, six of the double-linguists dropped French, so that now only a quarter of the French students study Spanish.  How many pupils are at the Euler Academy?”

Just to be clear, “French students” means Euler Academy pupils studying French, and similarly for “Spanish students.”

Answer.

See the Language Students Puzzle for solution.

Milk Mixing Puzzle

This is a classic example of a mixture problem from Dan Griller that recalls my agonies of beginning algebra.

“In Cauchy Village, full fat milk has 3.5% fat content, semi-skimmed milk has a 1.5% fat content, and skimmed milk has a 0.2% fat content. How many liters of full fat milk must be added to 100 liters of skimmed milk to produce semi-skimmed milk?”

Answer.

See the Milk Mixing Puzzle for solution.

Pool Paving Problem

In my search for problems I decided to purchase Dan Griller’s GCSE problem book  mentioned in the Cube Roots Problem.  I am still a bit confused about the purpose of the GCSE exam and who it is for, since the other problems in Griller’s book are often as challenging or more so than the cube roots problem.  It is hard to believe students not pursuing college level degrees could solve these problems.  (Grades 8 and 9 referred to in the subtitle of the book must indicate something other than US grades 8 and 9, since the exams are aimed at 16 year-olds, not 13 and 14 year-olds.)

Supposedly the problems in Griller’s book are nominally arranged in increasing order of difficulty from problem 1 to problem 75.  However it seemed to me that there were challenging problems scattered throughout and the last problem was not all that much harder than earlier ones.  And many of them had a whiff of Coffin Problems—they seemed impossible at first (Problem 44: Construct a 67.5° angle!).  I don’t know how many problems are on the exam or how long the exam is, but anyone taking a timed exam does not have the leisure to mull over a problem.  The student only has a few minutes to come up with an approach and clever insights are rare under the circumstances.  Anyway, here is the last problem in the book.

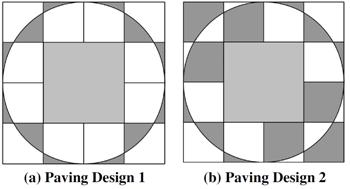
“Problem 75.  A square pond of side length 2 metres is to be surrounded by twelve square paving stones of side length 1 metre.

(a)  The first design is constructed with a circle whose centre coincides with the centre of the pond.  Calculate exactly the total dark grey area for this design.

(b)  The second design is similar.  Calculate exactly the total dark grey area for this second design.”

Answer.

See the Pool Paving Problem for solutions.

Cube Roots Problem

In a June Chalkdust book review of Daniel Griller’s second book, Problem solving in GCSE mathematics, Matthew Scroggs presented the following problem #65 from the book (without a solution):
“Solve _______________”

Scroggs’s initial reaction to the problem was “it took me a while to realise that I even knew how to solve it.”

Mind you, according to Wikipedia, “GCSEs [General Certificate of Secondary Education] were introduced in 1988 [in the UK] to establish a national qualification for those who decided to leave school at 16, without pursuing further academic study towards qualifications such as A-Levels or university degrees.” My personal feeling is that any student who could solve this problem should be encouraged to continue their education with a possible major in a STEM field.

Answer.

See Cube Roots Problem for a solution.