 This is an interesting algebra problem from BL’s Weekly Math Games, which is behind a subscription wall.
This is an interesting algebra problem from BL’s Weekly Math Games, which is behind a subscription wall.
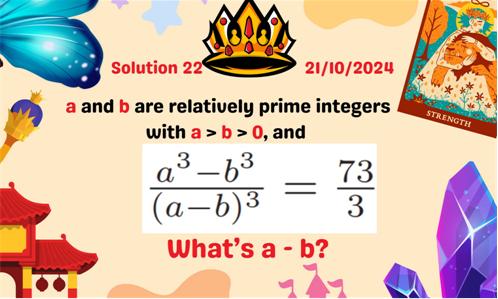
(a3 – b3)/(a – b)3 = 73/3,
what is a – b?”
In fact, it is possible to solve for a and b individually as well.
See a Tricky Ratio Puzzle for the solution.
(Updates 11/6/2024, 11/9/2024) Other Solutions
(Update 11/6/2024) BL’s Solution
Rummaging around I found the beginnings of BL’s solution, again because I am not a subscriber. I added the remaining steps as I imagined them. His solution is simpler.
See BL’s Solution
(Update 11/9/2024) Oscar Roja’s Solution
Oscar Rojas has a different take on a solution.

LHS = (a^3-b^3)/(a-b)^3
=(a^2 + a*b + b^2)/((a-b)^2
=(a^2 – 2*a*b + b^2 + 3*a*b)/((a-b)^2
=[ (a-b)^2 + 3*a*b]/(a-b)^2
= 1 + 3*a*b/(a-b)^2 = RHS = 73/3
3*a*b/(a-b)^2 = 73/3 -1 = 70/3
a*b / (a-b)^2 = 70/9
since 70 and 9 are relatively prime,
a*b = 70 and a-b = sqrt(9) = 3
a*b=70 can be expressed as
70*1 or 35*2 or 14*5 or 10*7
Only 10 and 7 gives a-b=10-7=3.
Hence a=10 and b=7
*************