This article is basically a technical footnote without wider significance. At the time I had been reading with interest Paul J. Nahin’s latest book Number-Crunching (2011). Nahin presents a problem that he will solve with the Monte Carlo sampling approach.

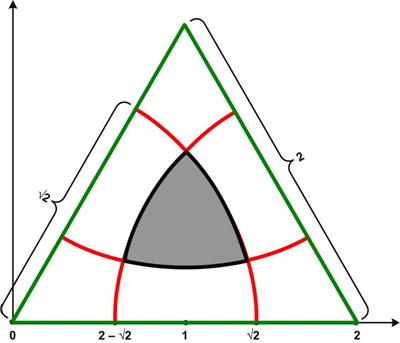
“To start, imagine an equilateral triangle with side lengths 2. If we pick a point ‘at random’ from the interior of the triangle, what is the probability that the point is no more distant than d = √2 from each of the triangle’s three vertices? The shaded region in the figure is where all such points are located.”

Nahin provided a theoretical calculation for the answer and said that it “requires mostly only high school geometry, plus one step that I think requires a simple freshman calculus computation.” This article presents my solution without calculus. See the Nahin Triangle Problem.