This is a collection of simple but elegant puzzles, mostly from a British high school math teacher Catriona Shearer, for which I thought I would show solutions (solutions for a number of them had not been posted yet on Twitter at the time of writing).  See the Geometric Puzzle Medley.

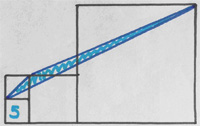
Apparently Catriona Shearer creates these problems herself, which shows an especially gifted talent. Ben Olin, of Math with Bad Drawings fame, had an interesting interview with Ms. Shearer. The reason for the interest in her work becomes evident the more of her geometry problems one sees. They are especially elegant and minimalist, and often have simple solutions, as exemplified by the “5 Problem” or “Shear Beauty” problem illustrated here. Words, such as “beauty” and “elegance”, are often bandied about concerning various mathematical subjects, but as with any discussion of esthetics, the efforts at explanation usually fall flat. Shearer’s problems are one of the best examples of these ideas I have ever seen. If you contemplate her problems and even solve them, you will understand the meaning of these descriptions.

One of the key aspects of mathematics is often its “hidden-ness” (some would say “opacity” or “incomprehension”). Her problems appear to have insufficient information to solve. But as you look at the usually regular figures, you see that there are inherent rigid constraints that soon yield specific information that leads to a solution. This discovery is akin to the sensation of discovering Newton’s mathematical laws underlying physical reality. It is the essence of one of the joys of mathematics.

This praise impinges on one of my soap-box subjects, which I won’t belabor here, namely, the value of plane geometry. I am no longer directly involved in public education, but every so often I see attacks on the mathematical curriculum centered on the requirement for plane geometry in high school. All the usual arguments over relevance and utility are brought to bear. But – and some day I may elaborate on why I became a mathematician – the only real mathematics I ever experienced in K-12 was in the plane geometry course. There I learned for the first time about proofs, reasoning, and a meaning of “truth” – an initial slaking of my thirst for answers to the many raging “whys” of a teenage mind. It was that course that led me to want to have mathematics play a central role in my life (after a frustrating detour through physics). One of my close mathematical friends also determined on a future in mathematics after his high school plane geometry course. Even for those not intending on a career in science, plane geometry is a very accessible way to grasp the fundamentals of a mathematical view of things. Furthermore, no educated person can understand the intellectual history of the West (and the many influences from the East) without appreciating the central role played by plane geometry for over 2000 years before the advent of symbolic algebra by the 16th century. Imagine what I would say if I were to belabor the issue!